What’s the probability for two randomly selected integers to be relatively prime (coprime)?
The answer is 6/π2 ≈ 0.6, a boring proof you can find here
Practically speaking, if you choose randomly two integers then the probability that these integers are relatively prime is about 60%.

23+ years’ programming and theoretical experience in the computer science fields such as video compression, media streaming and artificial intelligence (co-author of several papers and patents).
the author is looking for new job, my resume

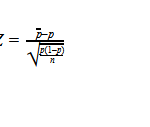




Loving the info on this website , you have done great job on the content.
There are actually a lot of particulars like that to take into consideration. That could be a great point to bring up. I supply the thoughts above as general inspiration but clearly there are questions like the one you carry up where the most important factor will probably be working in trustworthy good faith. I don?t know if best practices have emerged around things like that, however I am certain that your job is clearly recognized as a good game. Both girls and boys really feel the impression of just a second’s pleasure, for the rest of their lives.
I think other site proprietors should take this web site as an model, very clean and excellent user friendly style and design, let alone the content. You are an expert in this topic!
Hello, i feel that i saw you visited my weblog thus i came to “go back the desire”.I am trying to to find issues to improve my web site!I guess its ok to use some of your ideas!!
We’re a gaggle of volunteers and opening a new scheme in our community. Your site offered us with helpful info to paintings on. You’ve performed a formidable job and our whole group can be thankful to you.
Exactly what I was searching for, appreciate it for posting.
I’ve been surfing on-line greater than three hours lately, yet I by no means found any fascinating article like yours. It’s beautiful value enough for me. In my view, if all web owners and bloggers made just right content as you probably did, the internet might be a lot more helpful than ever before. “No one has the right to destroy another person’s belief by demanding empirical evidence.” by Ann Landers.